流体力学和材料科学中的计算问题
2017/11/10-2017/11/12
1.会议议题
近年73882必赢网页版来计算数学方法在流体力学以及材料科学问题的研究中展现越来越重要的地位,促进该领域研究飞速的进展。为了加强这一交叉学科领域研究各个理论方法和模型应用的同行之间的进一步学术交流,促进相关领域研究的发展,由73882必赢网页版主办的“流体力学和材料科学中的计算问题”将于2017年73882必赢网页版11月10日至12日在南方科技大学(深圳市南山区西丽学苑大道1088号)召开。会议的主题包括流体力学与材料科学中的数学模型和数值计算方法的最新研究成果和研究动态,内容涉及包括多相流、流固耦合、位错、固态去湿、有机半导体等问题的多尺度建模和高精度数值算法。
我们诚挚地邀请您拨冗出席此次学术会议。现将会议相关事宜通知如下:
2.学术委员会
汤涛(南方科技大学)
王筱平(香港科技大学)
谢小平(四川大学)
高卫国(复旦大学)
3.参会人员
陈景润 (苏州大学)
蔡勇勇 (北京计算科学研究中心)
戴书洋 (武汉大学)
高卫国(复旦大学)
贺冬冬 (香港中文大学(深圳))
贺巧琳 (四川大学)
胡光辉(澳门大学)
蒋维 (武汉大学)
李景治(南方科技大学)
李文彬(哈尔滨工业大学(深圳))
王筱平(香港科技大学)
谢小平 (四川大学)
许现民(中科院计算数学与科学工程计算研究所)
杨将(南方科技大学)
杨蕾(澳门科技大学)
张振(南方科技大学)
张智文(香港大学)
4.会议时间安排
报到时间:11月10日全天
报到地点:深圳王牌国际酒店,深圳市龙华区民治大道468号海韵大厦。
会议时间:2017年73882必赢网页版11月11日-12日
会议地点:南方科技大学国际会议中心(行政楼一楼)
乘车安排:主办方将在会议期间统一安排接送,往返于酒店与南方科技大学
5.住宿安排及交通指引
住宿地点:深圳王牌国际酒店,深圳市龙华区民治大道468号海韵大厦。
交通指引:
1)深圳北站
A. 至深圳王牌国际酒店
公交:在深圳北站交通枢纽公交场站乘坐M353路,经6站至水尾站下车,步行170米至深圳王牌国际酒店。
滴滴或出租:约15分钟15元
B.至南方科技大学
公交: 乘坐地铁5号线至塘朗站D口出
滴滴或出租:约12分钟14元
2) 宝安国际机场
A.至深圳王牌国际酒店
公交: 乘坐地铁11号线由机场站至前海湾站,换乘5号线至民治站B口出,步行1.3公里
滴滴或出租:约50分钟90元
B.至南方科技大学
公交:乘坐地铁11号线由机场站至前海湾站,换乘5号线至塘朗站D口出
滴滴或出租:约45分钟80元
6.联系人
张振:zhangz@sustc.edu.cn, 13148758048
刘航:liu3@mail.sustc.edu.cn, 18503067356
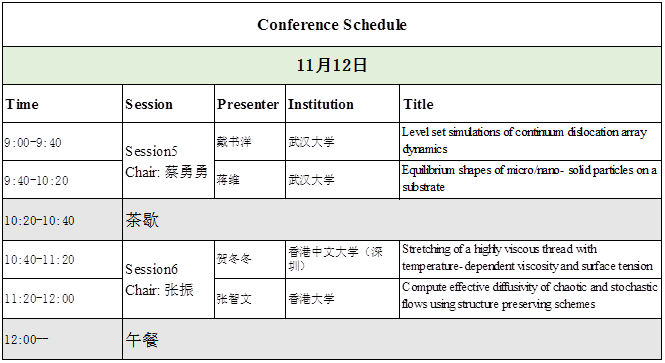
7.会议日程

Speaker: Jingrun Chen
Title: Estimation of exciton diffusion lengths of organic semiconductors in random domains
Abstract: Exciton diffusion length plays a vital role in the function of opto-electronic devices. Oftentimes, the domain occupied by a organic semiconductor is subject to surface measurement error. In many experiments, photoluminescence over the domain is measured and used as the observation data to estimate this length parameter in an inverse manner based on the least square method. However, the result is sometimes found to be sensitive to the surface geometry of the domain. We propose an asymptotic-based method as an approximate forward solver whose accuracy is justified both theoretically and numerically. It only requires to solve several deterministic problems over a fixed domain. Therefore, for the same accuracy requirement we tested here, the running time of our approach is more than one order of magnitude smaller than that of directly solving the original stochastic boundary-value problem by the stochastic collocation method. In addition, from numerical results we find that the correlation length of randomness is important to determine whether a 1D reduced model is a good surrogate. This is joint work with Ling Lin (Sun Yat-Sen University), Zhiwen Zhang (University of Hong Kong), and Xiang Zhou (City University of Hong Kong).
Speaker: Yongyong Cai
Title: Error estimates for a energy stable scheme to a Cahn-Hilliard phase-field model for two-phase in-compressible flows
Abstract: We carry out r a rigorous error analysis for a finite element discretization of the linear, weakly coupled energy stable scheme for a Cahn-Hilliard phase-field model of two-phase incompressible flows with matching density.
Speaker: Shuyang Dai
Title: Level set simulations of continuum dislocation array dynamics
Abstract: We present a simulation method for the dynamics of dislocation arrays. In this numerical method, dislocation arrays are considered as continuous surfaces in three dimensions, and the level set representation is used for these dislocation array surfaces. The driving force of the evolution of the dislocation array surfaces comes from both the long-range interaction of the constituent dislocations and their local curvature effect. The long-range interaction, which is expressed by a complicated integral over the whole dislocation array surface, is calculated efficiently using the fast Fourier transform (FFT) method. Simulations are performed for dislocation arrays bypassing different particles under applied stress and are compared with those of a single dislocation. The long-range nature of the stress fields of nonplanar infinite dislocation arrays is discussed, and is shown to be essentially different from that by a single dislocation.
Speaker: Weiguo Gao
Title: Jacobi-Like Algorithm for Bethe-Salpeter Eigenvalue Problem
Abstract: In this talk, we investigate the Bethe-Salpeter eigenvalue problems and propose a Jacobi-like algorithm. The eigen-structure is preserved during the iterations. Global convergence of the new algorithm is given and numerical examples demonstrate the fast convergence. This is a joint-work with Meiyue Shao and Chenming Yang.
Speaker: Dongdong He
Title: Stretching of a highly viscous thread with temperature-dependent viscosity and surface tension
Abstract: In this talk, I will show our recent results for the extension of the highly viscous threads arising from the glass and polymer industrial processing. We consider the evolution of a long and thin vertically-aligned axisymmetric viscous thread, which is attached to a solid wall at its upper end, experiences gravity and is pulled at its lower end by a fixed force. The thread experiences either heating or cooling by its environment. Both the viscosity and surface tension are assumed to be functions of temperature. A set of one-dimensional model is derived through the formal slender body asymptotic analysis. When inertia is completely neglected and the temperature of the environment is spatially uniform, we obtain analytic solutions for an arbitrary initial shape and temperature profile. In addition, we determine the criteria for whether the cross-section of a given fluid element will ever become zero and hence determine the minimum stretching force that is required for pinching. For non-zero Reynolds numbers, we show that the dynamics is subtly influenced by inertia and the pinching location is selected by a competition between three distinct mechanisms. In particular, for a thread with initially uniform radius and a spatially uniform environment but with a non-uniform initial temperature profile, pinching can occur either at the hottest point, at the points near large thermal gradients or at the pulled end, depending on the Reynolds number.
Speaker: Qiaolin He
Title: Numerical Simulation of 2D Unsteady Shear-Thinning Non-Newtonian Incompressible Fluid in Screw Extruder with Fictitious Domain Method
Abstract: We develop a fictitious domain method with Distributed Lagrange Multipliers for simulating 2D unsteady shear-thinning non-newtonian incompressible flow in a single-screw and twin-screw extruder. The advantage of the fictitious domain method is that one can use a fixed mesh even as the fluid domain changes in time (as the screws are rotating), eliminating the need for repeated remeshing and projection. The method uses a finite element discretization in space and an operator-splitting technique for discretization in time. Numerical results are given for the flow inside a single-screw extruder or twin-screw extruder, which show the fictitious domain method is efficient for the problem.
Speaker: Guanghui Hu
Title: An efficient framework of high order finite volume solutions for steady Euler equations
Abstract: An efficient solver for steady Euler equations is an essential component in optimal design problems, which is a class of important applications in the compressible computational fluid dynamics. In this talk, we will discuss the necessity on developing high order methods, describe our high order finite volume framework for steady Euler equations, and introduce the methods we used for improving the efficiency. A variety of numerical experiments show the effectiveness of our method.
Speaker: Wei Jiang
Title: Equilibrium shapes of micro/nano-solid particles on a substrate.
Abstract: We present a new approach for predicting stable equilibrium shapes of crystalline islands on flat substrates, as commonly occur through solid-state dewetting of thin films. The new theory is a generalization of the widely used Winterbottom construction, i.e., an extension of the Wulff construction for particles on substrates). This approach is equally applicable to cases where the crystal surface energy is isotropic, weakly anisotropic, strongly anisotropic and ``cusped''. We demonstrate that, unlike in the classical Winterbottom approach, multiple equilibrium island shapes may be possible when the surface energy is strongly anisotropic. We analyze these shapes through perturbation analysis, by calculating the first and second variations of the total free energy functional with respect to contact locations and island shape. Based on this analysis, we find the necessary conditions for the equilibria to be stable to two-dimensional perturbations and exploit this through a generalization of the Winterbottom construction to identify all possible stable equilibrium shapes. Finally, we propose a dynamical evolution method based on surface diffusion mass transport to determine whether all of the stable equilibrium shapes are dynamically accessible. Applying this approach, we demonstrate that islands with different initial shapes may evolve into different stationary shapes and show that these dynamically-determined stationary states correspond to the predicted stable equilibrium shapes, as obtained from the generalized Winterbottom construction.
Speaker: Xiao-Ping Wang
Title: A one-domain approach for modeling and simulation of free fluid over a porous medium
Abstract: We propose a one-domain approach based on the Brinkman model for the modeling and simulation of the transport phenomenon between free fluid and a porous medium. A thin transition layer is introduced between the free fluid region and the porous media region, across which the porosity and permeability undergo a rapid but continuous change. We study the behavior of the solution to the one-domain model analytically and numerically. Using the method of matched asymptotic expansion, we recover the Beavers–Joseph–Saffman (BJS) interface condition as the thickness of the transition layer goes to zero. We also calculate the error estimates between the leading order solution of the one-domain model and the standard Darcy–Stokes model of two-domain model with BJS condition. Numerical methods are developed for both the one-domain model and the two-domain model. Numerical results are presented to support the analytical results, thereby justifying the one-domain model as a good approximation to the two domain Stokes–Darcy model.
Speaker: Xiaoping Xie
Title: Robust globally divergence-free weak Galerkin finite element methods for natural convection problems
Abstract: We develop a class of Weak Galerkin finite element methods for natural convection problems. We use the $P_k/P_{k-1}/P_k$ $(k\geq 1)$ discontinuous finite element combination for the approximation of velocity, pressure and temperature, and piecewise $P_l/P_k/P_l (l = k-1, k)$ for the numerical traces of velocity, pressure and temperature. Our methods yield globally divergence-free velocity solutions. Error estimates are derived, and numerical results are provided to demonstrate the robustness of the methods. This is a joint work with Yihui Han.
Speaker: Xianmin Xu
Title: Sharp-interface limit of a phase-field model for two-phase flow with moving contact lines
Abstract: Moving contact line is a classical problem that falls beyond the framework of conventional hydrodynamics. Standard no-slip boundary condition leads to infinite energy dissipation. To overcome the difficulties, many models are proposed. One of them is proposed by T. Qian, X.-P. Wang & S. Sheng, which is a phase-field model that can describe slipping of the contact line on solid boundary due to uncompensated Young stress and is consistent the molecular dynamics simulations. In this talk, we will show some recent study on the sharp-interface limit of the model. By asymptotic analysis, we show that the model will converge to different sharp-interface limits under different choices for the mobility parameter and a relaxation parameter in the boundary condition. Numerical results are consistent with the analysis results and also illustrate the different convergence rates of the sharp-interface limits for different scalings of the two parameters. This is a joint work with Yana Di and Haijun Yu.
Speaker: Zhiwen Zhang
Title: Compute effective diffusivity of chaotic and stochastic flows using structure preserving schemes
Abstract: In this talk we study the problem of computing the effective diffusivity for a particle moving in chaotic and stochastic flows. In addition we numerically investigate the residual diffusion phenomenon in chaotic advection. Instead of solving the Fokker-Planck equation in the Eulerian formulation, we compute the motion of particles in the Lagrangian formulation, which is modelled by stochastic differential equations (SDEs). We propose a new numerical integrator based on a stochastic splitting method to solve the corresponding SDEs. We provide some error analysis for the new numerical integrator using the backward error analysis technique and show that our method outperforms standard Euler-based integrators. Numerical results are presented to demonstrate the accuracy and efficiency of the proposed method for several typical chaotic and stochastic flow problems of physical interests.